Search any question & find its solution
Question:
Answered & Verified by Expert
3 moles of an ideal monoatomic gas performs $A B C D A$ cyclic process as shown in figure below. The gas temperatures are $T_A=400 \mathrm{~K}$, $T_B=800 \mathrm{~K}, T_C=2400 \mathrm{~K}$ and $T_D=1200 \mathrm{~K}$. The work done by the gas is (approximately) $(R=8.314 \mathrm{~J} / \mathrm{mol} \mathrm{K})$
Options:

Solution:
2454 Upvotes
Verified Answer
The correct answer is:
$20 \mathrm{~kJ}$
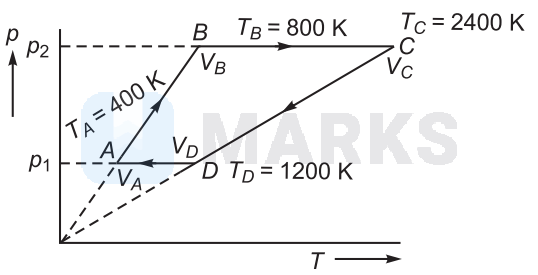
Processes $A$ to $B$ and $C$ to $D$ are parts of straight line graphs of form $y=m x$.
Also,
$p=\frac{\mu R}{V} T(\mu=3)$
$p \propto T$
So, volume remains constant for the graphs $A B$ and $C D$.
So, no work is done during processes for $A$ to $B$ and $C$ to $D$.
$W_{A B}=W_{C D}=0$
and $\quad W_{B C}=p_2\left(V_C-V_B\right)$
$=\mu R\left(T_C-T_B\right)$
$=3 R(2400-800)$
$=3 R \times 1600$
$=4800 R$
$W_{D A}=p_1\left(V_A-V_D\right)$
$=\mu R\left(T_A-T_D\right)$
$=3 R(400-1200)$
$=-2400 R$
Work done in complete cycle
$W=W_{A B}+W_{B C}+W_{C D}+W_{D A}$
$=0+4800 R+0+(-2400) R$
$=2400 R$
$=19.944 \mathrm{~J}=20 \mathrm{~kJ}$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.