Search any question & find its solution
Question:
Answered & Verified by Expert
A 4 kg block is suspended from the ceiling of an elevator through a spring having a linear mass density of $19.2 \times 10^{-3} \mathrm{~kg} \mathrm{~m}^{-3}$. Find the speed with respect to spring with which a wave pulse can proceed on the spring if the elevator accelerates up at the rate of $2.0 \mathrm{~ms}^{-2}$. Take $g=10 \mathrm{~ms}^{-2}$
Options:
Solution:
1726 Upvotes
Verified Answer
The correct answer is:
50 m/s
Given, $m=19.2 \times 10^{-3} \mathrm{~kg} / \mathrm{m}$
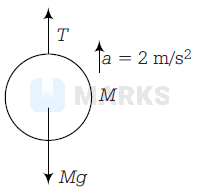
Now see the free body diagram.
The equation for the motion
$\begin{aligned} T-4 g-4 a & =0 \\ T & =4(a+g)=4(2+10) \\ & =48 \mathrm{~N}\end{aligned}$
Now wave speed,
$\begin{aligned} v & =\sqrt{\left(\frac{T}{m}\right)} \\ & =\sqrt{2.5 \times 10^{-3}}=50 \mathrm{~m} / \mathrm{s}\end{aligned}$
Now see the free body diagram.
The equation for the motion
$\begin{aligned} T-4 g-4 a & =0 \\ T & =4(a+g)=4(2+10) \\ & =48 \mathrm{~N}\end{aligned}$

Now wave speed,
$\begin{aligned} v & =\sqrt{\left(\frac{T}{m}\right)} \\ & =\sqrt{2.5 \times 10^{-3}}=50 \mathrm{~m} / \mathrm{s}\end{aligned}$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.