Search any question & find its solution
Question:
Answered & Verified by Expert
A current \(I\) a flows in a circular arc of radius \(r\) subtending an angle \(\theta\) as shown in the figure. Find the magnetic field at the centre \(O\) of the circle.
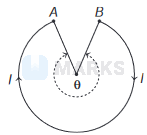
Options:

Solution:
2397 Upvotes
Verified Answer
The correct answer is:
\(\frac{\mu_0 I \theta}{4 \pi r}\)
Magnetic field at the centre due to current carrying circular loop is given as
\(B_C=\frac{\mu_0 I}{2 r}\) ...(i)
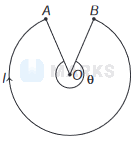
Magnetic field due to current carrying circular arc making an angle \(\theta\) at the centre, is given as
\(\begin{aligned}
& B=\frac{\theta}{2 \pi} \cdot B_C=\frac{\theta}{2 \pi} \cdot \frac{\mu_0 I}{2 r} \text { [From Eq. (i)] } \\
& B=\frac{\mu_0 I \theta}{4 \pi r}
\end{aligned}\)
\(B_C=\frac{\mu_0 I}{2 r}\) ...(i)

Magnetic field due to current carrying circular arc making an angle \(\theta\) at the centre, is given as
\(\begin{aligned}
& B=\frac{\theta}{2 \pi} \cdot B_C=\frac{\theta}{2 \pi} \cdot \frac{\mu_0 I}{2 r} \text { [From Eq. (i)] } \\
& B=\frac{\mu_0 I \theta}{4 \pi r}
\end{aligned}\)
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.