Search any question & find its solution
Question:
Answered & Verified by Expert
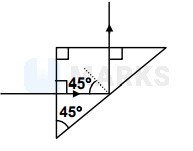
A light ray is incident perpendicular to one face of a $90^{\circ}$ prism and is totally internally reflected at the glass-air interface. If the angle of reflection is $45^{\circ}$, we conclude that the refractive index $n$
Options:

Solution:
2612 Upvotes
Verified Answer
The correct answer is:
$\mathrm{n}>\sqrt{2}$
$\mathrm{n}>\sqrt{2}$
Angle of incidence $\mathrm{i}>\mathrm{C}$ for total internal reflection. Here $\mathrm{i}=45^{\circ}$ inside the medium.
$$
\begin{aligned}
& \therefore 45^{\circ}>\sin ^{-1}(1 / n) \\
& \Rightarrow n>\sqrt{ } 2 .
\end{aligned}
$$
$$
\begin{aligned}
& \therefore 45^{\circ}>\sin ^{-1}(1 / n) \\
& \Rightarrow n>\sqrt{ } 2 .
\end{aligned}
$$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.