Search any question & find its solution
Question:
Answered & Verified by Expert
The magnetic moment $\left(\mathrm{m}_{\mathrm{orb}}\right)$ of a revolving electron around the nucleus varies with the principal quantum number (n) as
Options:
Solution:
1032 Upvotes
Verified Answer
The correct answer is:
$\mathrm{m}_{\text {orb }} \propto \mathrm{n}$
Orbital magnetic moment can be defined as,
$\mathrm{m}_{\text {orb }}=\mathrm{iA}$
where, $\mathrm{i}=\frac{\mathrm{e}}{\mathrm{T}}, \mathrm{r}$ is the radius of the Bohr orbit, $\mathrm{A}=\pi \mathrm{r}^2$ is the area and $\mathrm{T}$ is the time period of uniform circular motion.

Now, we can make use of the Bohr's hypothesis about angular momentum:
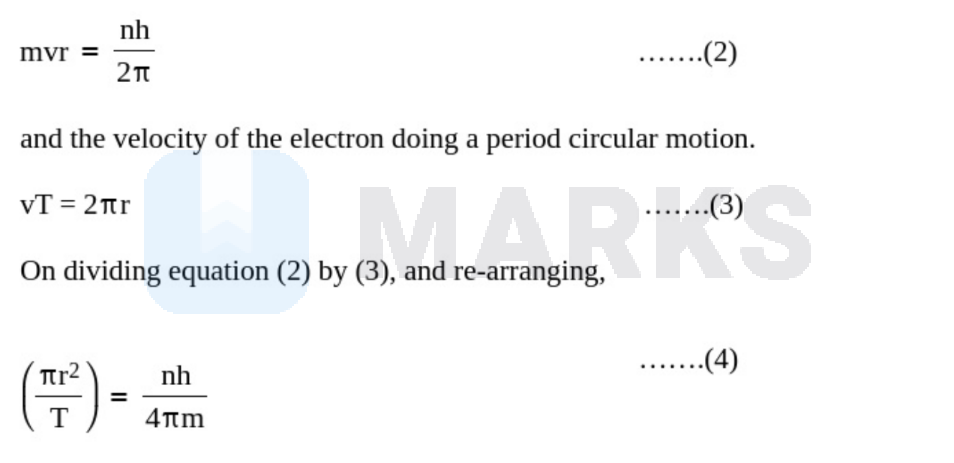
On plugging in above into equation (1),
$\mathrm{m}_{\mathrm{orb}}=\frac{\mathrm{neh}}{4 \pi \mathrm{m}}$
Orbital magnetic moments of an electron in Bohr orbit is given by,
$\begin{aligned} & \mathrm{m}_{\text {orb }}=\mathrm{n}\left(\frac{\mathrm{eh}}{4 \pi \mathrm{m}}\right) \\ & \therefore \mathrm{m}_{\text {orb }} \propto \mathrm{n}\end{aligned}$
$\mathrm{m}_{\text {orb }}=\mathrm{iA}$
where, $\mathrm{i}=\frac{\mathrm{e}}{\mathrm{T}}, \mathrm{r}$ is the radius of the Bohr orbit, $\mathrm{A}=\pi \mathrm{r}^2$ is the area and $\mathrm{T}$ is the time period of uniform circular motion.

Now, we can make use of the Bohr's hypothesis about angular momentum:

On plugging in above into equation (1),
$\mathrm{m}_{\mathrm{orb}}=\frac{\mathrm{neh}}{4 \pi \mathrm{m}}$
Orbital magnetic moments of an electron in Bohr orbit is given by,
$\begin{aligned} & \mathrm{m}_{\text {orb }}=\mathrm{n}\left(\frac{\mathrm{eh}}{4 \pi \mathrm{m}}\right) \\ & \therefore \mathrm{m}_{\text {orb }} \propto \mathrm{n}\end{aligned}$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.