Search any question & find its solution
Question:
Answered & Verified by Expert
The position vectors of $A$ and $B$ are $(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})$ and $\left.\left(\frac{1}{3} \hat{\mathbf{j}}+\frac{1}{3} \hat{\mathbf{k}}\right)\right)$. If $B$ divides the line $A C$ in the ratio $2: 1$, then position vector $C$ is
Options:
Solution:
2524 Upvotes
Verified Answer
The correct answer is:
$\left(\frac{-1}{2}, 0,0\right)$
Let $\mathbf{O A}=(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})$ and $\mathbf{O B}=\left(\frac{1}{3} \hat{\mathbf{j}}+\frac{1}{3} \hat{\mathbf{k}}\right)$
Given ratio = 2 : 1
Let m: n = 2:1
m = 2and n = 1
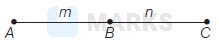
$\begin{aligned} & \because \mathbf{O B}=\frac{m \mathbf{O C}+n \mathbf{O A}}{m+n}=\frac{2 O C+(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})}{3} \\ & 3\left(\frac{1}{3} \hat{\mathbf{j}}+\frac{1}{3} \hat{\mathbf{k}}\right)=2(\text { OC })+(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}) \\ & \hat{\mathbf{j}}+\hat{\mathbf{k}}-\hat{\mathbf{i}}-\hat{\mathbf{j}}-\hat{\mathbf{k}}=2 \cdot(\text { OC }) \\ & \Rightarrow \quad \text { OC }=\frac{-1}{2} \hat{\mathbf{i}}+0 \hat{\mathbf{j}}+0 \hat{\mathbf{k}} \\ & \therefore \quad C=\left(\frac{-1}{2}, 0,0\right) \\ & \end{aligned}$
Given ratio = 2 : 1
Let m: n = 2:1
m = 2and n = 1
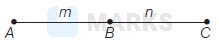
$\begin{aligned} & \because \mathbf{O B}=\frac{m \mathbf{O C}+n \mathbf{O A}}{m+n}=\frac{2 O C+(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}})}{3} \\ & 3\left(\frac{1}{3} \hat{\mathbf{j}}+\frac{1}{3} \hat{\mathbf{k}}\right)=2(\text { OC })+(\hat{\mathbf{i}}+\hat{\mathbf{j}}+\hat{\mathbf{k}}) \\ & \hat{\mathbf{j}}+\hat{\mathbf{k}}-\hat{\mathbf{i}}-\hat{\mathbf{j}}-\hat{\mathbf{k}}=2 \cdot(\text { OC }) \\ & \Rightarrow \quad \text { OC }=\frac{-1}{2} \hat{\mathbf{i}}+0 \hat{\mathbf{j}}+0 \hat{\mathbf{k}} \\ & \therefore \quad C=\left(\frac{-1}{2}, 0,0\right) \\ & \end{aligned}$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.