Search any question & find its solution
Question:
Answered & Verified by Expert
The resistance of an ammeter is $13 \Omega$ and its scale is graduated for a current upto 100 A . After an additional shunt has been connected to this ammeter it becomes possible to measure currents upto 750 A by this meter. The value of shunt resistance is
Options:
Solution:
2244 Upvotes
Verified Answer
The correct answer is:
$2 \Omega$
Let $i_a$ is the current flowing through ammeter and $i$ is the total current. So, a current $i-i_a$ will flow through shunt resistance.
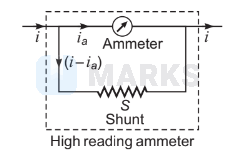
Potential difference across ammeter and shunt resistance is same.
ie, $\quad i_a \times R=\left(i-i_a\right) \times S$
or
$S=\frac{i_a R}{i-i_a}\ldots(i)$
Given, $\quad i_a=100 \mathrm{~A}, i=750 \mathrm{~A}, R=13 \Omega$
Hence, $\quad S=\frac{100 \times 13}{750-100}=2 \Omega$

Potential difference across ammeter and shunt resistance is same.
ie, $\quad i_a \times R=\left(i-i_a\right) \times S$
or
$S=\frac{i_a R}{i-i_a}\ldots(i)$
Given, $\quad i_a=100 \mathrm{~A}, i=750 \mathrm{~A}, R=13 \Omega$
Hence, $\quad S=\frac{100 \times 13}{750-100}=2 \Omega$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.