Search any question & find its solution
Question:
Answered & Verified by Expert
The shortest distance between the skew lines $\mathbf{r}=(6 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}})+t(\hat{\mathbf{i}}-2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}})$ and $\mathbf{r}=(-4 \hat{\mathbf{i}}-\hat{\mathbf{k}})+s(3 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}})$ is
Options:
Solution:
1017 Upvotes
Verified Answer
The correct answer is:
9
Given,
$\begin{array}{ll} & \mathbf{r}=(6 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}})+t(\hat{\mathbf{i}}-2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}}) \\ \text { and } & \mathbf{r}=(-4 \hat{\mathbf{i}}-\hat{\mathbf{k}})+s(3 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}) \\ \text { Here, } & \mathbf{a}=6 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}}, \mathbf{b}=\hat{\mathbf{i}}-2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}} \\ & \mathbf{c}=-4 \hat{\mathbf{i}}-\hat{\mathbf{k}}, \mathbf{d}=3 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}\end{array}$
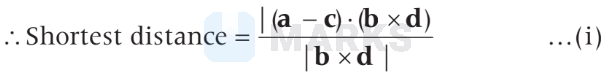
$$
\begin{aligned}
& \text { Now, } \quad \mathbf{b} \times \mathbf{d}=\left|\begin{array}{ccc}
\hat{\mathbf{i}} & \hat{\mathbf{j}} & \hat{\mathbf{k}} \\
1 & -2 & 2 \\
3 & -2 & -2
\end{array}\right| \\
& =\hat{\mathbf{i}}(4+4)-\hat{\mathbf{j}}(-2-6)+\hat{\mathbf{k}}(-2+6)=8 \hat{\mathbf{i}}+8 \hat{\mathbf{j}}+4 \hat{\mathbf{k}}
\end{aligned}
$$
By Eq. (i),
$$
\begin{aligned}
& =\left|\frac{(10 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+3 \hat{\mathbf{k}}) \cdot(8 \hat{\mathbf{i}}+8 \hat{\mathbf{j}}+4 \hat{\mathbf{k}})}{\sqrt{64+64+16}}\right| \\
& =\left|\frac{80+16+12}{\sqrt{144}}\right|=\frac{108}{12}=9
\end{aligned}
$$
$\begin{array}{ll} & \mathbf{r}=(6 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}})+t(\hat{\mathbf{i}}-2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}}) \\ \text { and } & \mathbf{r}=(-4 \hat{\mathbf{i}}-\hat{\mathbf{k}})+s(3 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}) \\ \text { Here, } & \mathbf{a}=6 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}}, \mathbf{b}=\hat{\mathbf{i}}-2 \hat{\mathbf{j}}+2 \hat{\mathbf{k}} \\ & \mathbf{c}=-4 \hat{\mathbf{i}}-\hat{\mathbf{k}}, \mathbf{d}=3 \hat{\mathbf{i}}-2 \hat{\mathbf{j}}-2 \hat{\mathbf{k}}\end{array}$
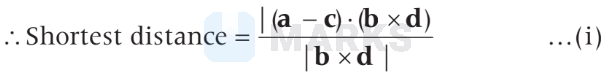
$$
\begin{aligned}
& \text { Now, } \quad \mathbf{b} \times \mathbf{d}=\left|\begin{array}{ccc}
\hat{\mathbf{i}} & \hat{\mathbf{j}} & \hat{\mathbf{k}} \\
1 & -2 & 2 \\
3 & -2 & -2
\end{array}\right| \\
& =\hat{\mathbf{i}}(4+4)-\hat{\mathbf{j}}(-2-6)+\hat{\mathbf{k}}(-2+6)=8 \hat{\mathbf{i}}+8 \hat{\mathbf{j}}+4 \hat{\mathbf{k}}
\end{aligned}
$$
By Eq. (i),
$$
\begin{aligned}
& =\left|\frac{(10 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+3 \hat{\mathbf{k}}) \cdot(8 \hat{\mathbf{i}}+8 \hat{\mathbf{j}}+4 \hat{\mathbf{k}})}{\sqrt{64+64+16}}\right| \\
& =\left|\frac{80+16+12}{\sqrt{144}}\right|=\frac{108}{12}=9
\end{aligned}
$$
Looking for more such questions to practice?
Download the MARKS App - The ultimate prep app for IIT JEE & NEET with chapter-wise PYQs, revision notes, formula sheets, custom tests & much more.